
After all, whatever we raise to power 0, we get 1.

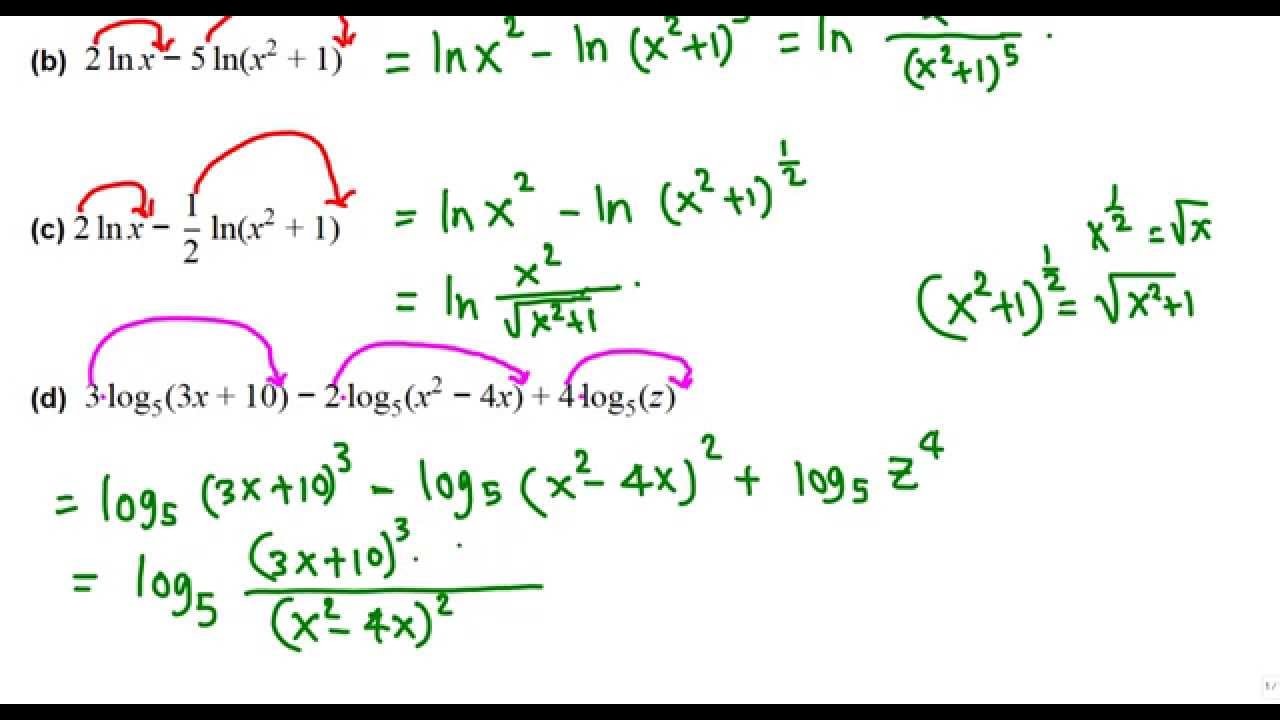
We denote them ln(x) and log(x) (the second one simply without the small 10), and their bases are, respectively, the Euler number e and (surprise, surprise!) the number 10. There are two very special cases of the logarithm which have unique notation: the natural logarithm and the logarithm with base 10.
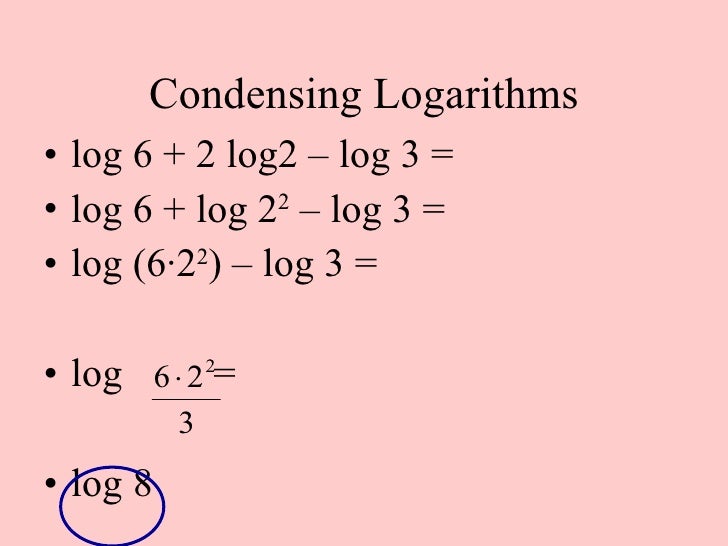
Condense the logarithm how to#
For example: ³√64 = 4īefore we learn how to rewrite logs, let's mention a few critical facts concerning them. However, as opposed to logarithms, roots return the exponent base, not the exponent itself (in the above language: they return how many people get infected by a single person). Note that taking a root is also considered an inverse operation to taking a power. In the above epidemic example, the logarithm (with base 4) returns at which step we get a fixed number of infected. Note, however, that in general, this can be (calc:1503). 💡 logₐ(b) gives you the power to which you'd need to raise a in order to obtain b. To make it all precise, let's see the following log definition. The logarithm is the inverse function to the exponential one. In general, we describe the number of sick in the n-th step by the exponent 4ⁿ. In turn, they got 4² = 4 * 4 = 16 people infected, who later got 4³ = 4 * 4 * 4 = 64 people infected.
Indeed, if we assume that each infected person transmits the disease onto, say, 4 others, then patient zero got 4 people sick. Mathematically speaking, such a thing is called exponential growth.Īs you might have noticed, the name suggests that it has something to do with exponents. With the COVID-19 pandemic raging on, there's been quite some talk about the rate at which the number of cases increases over time.


 0 kommentar(er)
0 kommentar(er)
